Engineers and designers use several analytic tools to understand behavior and characteristics. Sophisticated numerical techniques are used to make analysis and estimations easier for projects. Popularly used techniques for fluid analysis are the Finite Element Method (FEM) and the Finite Volume Method (FVM). Let’s explore the key differences in finite element vs finite volume method.

Immense investments and research have explored FEM and FVM and their contributions to Computational Fluid Dynamics (CFD) methods. Based on these studies, it is reported that there is no best method when comparing the two: fem vs fvm.

FEM vs FVM have various methods, and sometimes these methods overlap. The method’s efficiency or performance depends on the computational software and its practical application.

However, one can define the efficiency and superiority of FEM or FVM for a specific problem. Hence, it is vital to understand the difference between fem and fvm, and these techniques can help to determine which technique must be used where.

FEM vs FVM: What is the Finite Element Method?

The finite element method (FEM) is a numerical method to solve engineering, mathematical, or physics problems. The problems are in PDEs broken down into simpler equations and then translated systemically.

FEM is usually used to analyze heat transfer, structural analysis, fluid flow, electromagnetic potential, and mass transport. The problems are usually solved based on the boundary values.

FEM solves a system of algebraic problems broken down from a PDE. It also appraises the unknown function over the domain. Hence, FEM divides a big equation into smaller segments called finite elements or a node. Once all the finite elements are solved, they are combined to form a larger equation, which can now be solved quickly.

Advantages and Challenges of FEM (FEM vs FVM/Finite Element Method vs Finite Volume Method)

FEM is a general method that can be successfully used for Multiphysics analysis. FEM is already used to study electromagnetics and structural analysis.

This is generally used for locally approximating physics fields with higher polynomials. FEM caters to a natural increase in the order of elements that enables very accurate approximations in the physics fields.

FEM is also widely used to combine multiple functions with an element that helps in the estimation of the solution.

The drawback of FEM is that the mathematics used in this technique is advanced and cannot be performed without a FEM expert like Mechartes. Moreover, to maintain efficiency, time-dependent simulations use explicit solvers to make the FEM technique complicated. Hence, it is not advised in this case.

What is the FVM / Finite Volume Method?

The Finite Volume Method is a numerical technique widely used because of its fundamental conservation property. It involves evaluating volumes at hidden segments in a meshed geometry.

In FVM, the PDEs are converted into linear algebraic equations. Then, the approximation procedure is carried out in two basic steps.

i). The PDEs are transformed and integrated into balanced equations over an element. The surface and volume integrals are converted into discrete algebraic relations over elements and the surfaces with the help of an integration quadrature of a specified order of accuracy. This results in a set of semi-discrete equations.

ii). The variation in the variables in the elements is estimated by choosing the interpolation profiles. This is then related to the surface variables, which transform the algebraic relations into algebraic equations.

The approximation selections in FVM affect the overall accuracy of the subsequent numeric.

Advantages and Challenges of FVM (FEM vs FVM/Finite Element Method vs Finite Volume Method)

FVM is usually chosen to solve CFD problems as they involve conservation laws. FVM only needs to perform flux evaluation of the boundaries, making it an excellent choice for abiding by conservation laws.

Higher-order elements cannot be solved using FVM. However, the corner of interest can be accurately examined by refining the corner’s mesh.

What Should be Used Where?

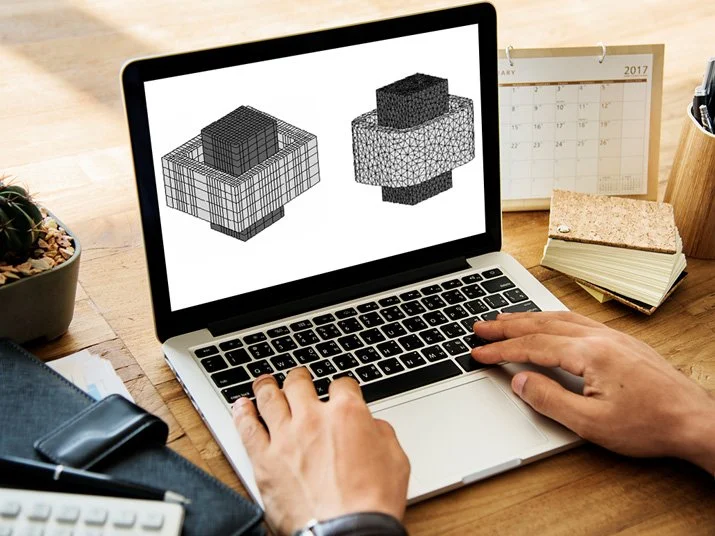
Both FEM and FVM are systemic numerical techniques used to solve PDEs. The only way engineers determine where to use Finite Element and Finite Volume is based on ease of use and implementation.

Engineers debate the sustainability of FEM for CFD analysis, and some believe that FVM is superior to FEM. However, the lack of corroborative evidence for this debate simply leaves the choice of methods up to the users.

The advantages and disadvantages of each method are used to determine which method must be used in a particular scenario.

As FEM vs FVM is being extensively used with CFD, understanding the pros and cons of each technique leads to a better estimation of the characteristics and features of the model in question.

With accurately fed data and industry knowledge, Mechartes can understand the model and perform analyses using the books. This expert guidance comes in handy for various projects. For more information, contact us today.